FRP pipe burst pressure is a critical aspect in determining the safety and performance of fiberglass-reinforced plastic piping systems. The frp pipe burst pressure barlows formula is commonly used to calculate the maximum pressure that an FRP pipe can withstand before failure. This calculation is essential for ensuring that pipes in industrial applications can handle operational pressures without risking damage or rupture. Barlow’s Formula relates the pipe’s wall thickness, allowable stress, and outside diameter to its burst pressure. The associated pipe burst pressure barlows formula will determine calculate an FRP pipe can withstand before failure. GangLong Fiberglass, a leading provider of FRP pipe, often utilizes the frp pipe burst pressure barlows formula to guarantee the strength and reliability of their products in various sectors, such as chemical processing and infrastructure projects. Understanding this calculation ensures safe design and operation.
FRP Pipe Burst Pressure Barlows Formula: A Comprehensive Guide
When dealing with fiberglass-reinforced plastic (FRP) piping systems, understanding the limitations and potential failure points is essential. One of the most critical factors is the burst pressure of the pipe, which can be effectively calculated using Barlow’s Formula. The frp pipe burst pressure barlows formula plays an important role in determining the maximum pressure an FRP pipe can handle before it ruptures. This guide will explore the formula, its variables, and its application in various industrial contexts.
The Key of Barlow’s Formula for FRP Pipe Burst Pressure
Barlow’s Formula is widely used in the piping industry to calculate burst pressure, and it’s highly relevant when it comes to FRP pipes. The frp pipe burst pressure barlows formula is expressed as:
P = (2 * T * S) / OD
Where:
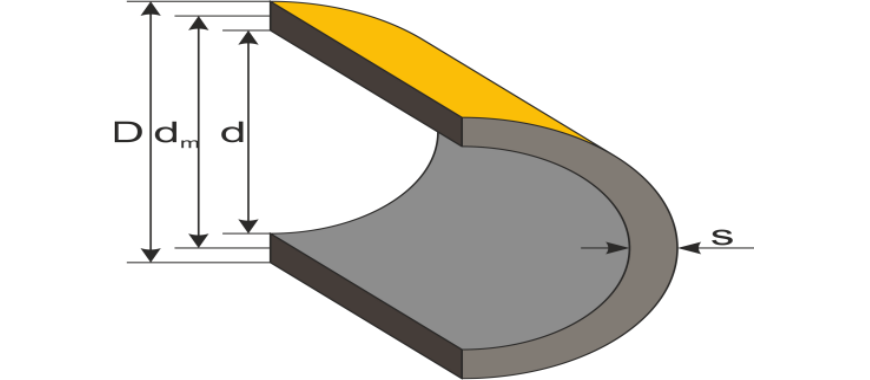
- P = Burst Pressure (the internal pressure at which the pipe will fail)
- T = Wall Thickness of the pipe
- S = Allowable Stress of the material
- OD = Outside Diameter of the pipe
This equation demonstrates how the burst pressure is directly influenced by the pipe’s wall thickness and allowable stress, while the outside diameter inversely affects the pressure tolerance. This formula is crucial for industries using FRP piping systems in environments where high pressure is present. GangLong Fiberglass frequently implements this formula in the design and production of its FRP pipes to ensure reliability.
How Barlow’s Formula Works in Real-World Applications
The frp pipe burst pressure barlows formula provides engineers with the ability to predict a pipe’s failure point under internal pressure. This predictive ability is important across various industries, including chemical processing, oil and gas, and water treatment. FRP pipes are known for their resistance to corrosion and lightweight properties, but even these advanced materials have limits, and that’s where Barlow’s Formula comes in.
For example, consider a GangLong Fiberglass FRP pipe with a wall thickness of 0.25 inches, an allowable stress of 10,000 psi, and an outside diameter of 12 inches. Using the frp pipe burst pressure barlows formula, you can calculate the burst pressure:
P = (2 * 0.25 * 10,000) / 12
P = 416.67 psi
This result shows that the pipe can withstand up to 416.67 psi of internal pressure before it is at risk of bursting. Adjusting any of these factors—whether the wall thickness, allowable stress, or outside diameter—will result in different burst pressures, demonstrating the flexibility and utility of Barlow’s Formula in real-world applications.
Importance of Wall Thickness in FRP Pipe Burst Pressure Calculations
One of the most significant variables in the frp pipe burst pressure barlows formula is the wall thickness. The thicker the pipe wall, the higher the burst pressure it can handle. This relationship is critical for industries where high-pressure fluids or gases are transported, as selecting the right wall thickness can prevent catastrophic failures.
In FRP piping systems, the wall thickness is influenced by various factors, including the diameter of the pipe, the pressure it is expected to handle, and the type of material used in its construction. For instance, GangLong Fiberglass offers a wide range of FRP pipes with different wall thicknesses, tailored to meet specific operational needs. Calculating the appropriate wall thickness using Barlow’s Formula is an essential step in the design process to ensure the pipe’s safety under pressure.
Derivation of FRP Pipe Burst Pressure with Barlows
The derivation of Barlow’s formula involves the application of basic principles of mechanics of materials, specifically focusing on the stress distribution in a thin-walled cylindrical vessel subjected to internal pressure. The formula estimates the internal pressure that would cause the cylinder to fail or burst. Below is a step-by-step derivation:
Assumptions
To begin with, several assumptions are made:
- The cylinder is thin-walled, meaning the wall thickness ttt is much smaller than the radius of the cylinder R(t≪R).
- The material of the cylinder is homogenous, isotropic, and elastic.
- The internal pressure is uniform across the cross-section.
- The cylinder is subjected only to internal pressure, and external forces or moments are neglected.
- The cylinder is assumed to be perfectly circular and smooth, without any imperfections.
Step 1: Define the Variables
Let’s define the key variables:
- P: Internal pressure inside the cylinder (force per unit area).
- R: Radius of the cylinder (in the plane of the cross-section).
- t: Thickness of the cylindrical wall.
- σ: Circumferential or hoop stress (the stress acting in the direction around the circumference of the cylinder).
- σlongitudinal: Longitudinal stress (the stress acting along the length of the cylinder).
Step 2: Apply Equilibrium and Stress Analysis
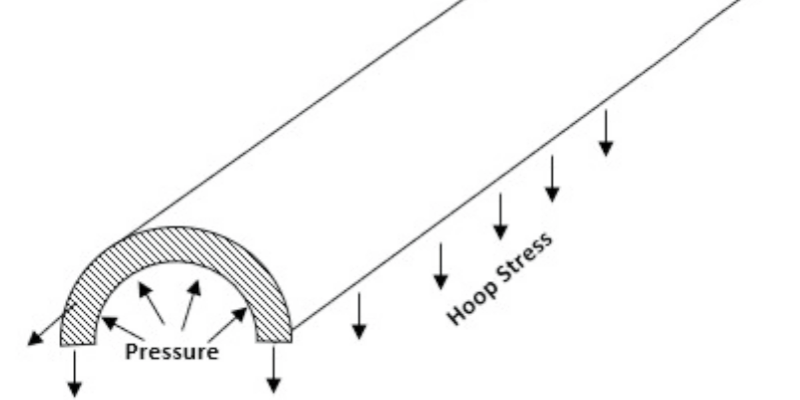
Consider a cylindrical segment of length LLL, with an internal radius RRR and a wall thickness ttt. This segment is subjected to an internal pressure PPP.
- The internal pressure creates a force acting radially outward on the surface of the cylinder.
- Due to this radial force, stresses develop in the material of the cylinder. The primary stress of concern in a pressurized cylinder is the hoop stress, which acts tangentially around the circumference of the cylinder.
The equilibrium condition for a thin-walled cylinder requires the balance of forces in the radial direction. The radial stress at the internal surface of the cylinder (r = R) is equal to the internal pressure PPP. Additionally, since the cylinder wall is thin, the radial stress can be approximated as negligible beyond the surface of the cylinder.
Step 3: Derive the Hoop Stress
For a thin-walled cylinder, the hoop stress σθ\sigma_{\theta}σθ, which is the circumferential stress, is the most critical stress. It is derived from the balance of forces in the radial direction as follows:
The internal force due to the pressure on the cylindrical surface is:
Finternal=P×Area=P×2πRL
Where 2πRL is the surface area of the cylinder over which the pressure acts.
Now, consider the equilibrium of the cylindrical segment. The force that resists the internal pressure is generated by the hoop stress acting along the circumference of the cylinder. The resisting force due to hoop stress is:
Fresisting=σθ×Area=σ
Where t×L is the cross-sectional area of the cylinder wall.
For equilibrium, these two forces must be equal:P×2πRL=σθ×t×L
Simplifying:P×2πR=σθ×tP \times 2\pi R = \sigma_{\theta} \times tP×2πR=σθ×t
Solving for the hoop stress σθ:
σθ=(P×2πR)/t
Step 4: Derive the Longitudinal Stress
The longitudinal stress σz\sigma_{z}σz (the stress along the length of the cylinder) can also be derived using similar principles. For a thin-walled cylinder under internal pressure, the longitudinal stress is half the hoop stress:
σz=σθ/2=P×2πR/2t=PπR/t
Step 5: Barlow’s Formula for Burst Pressure
Now, the burst pressure of the cylinder is the internal pressure PPP at which the hoop stress reaches the material’s yield or tensile strength. Assuming the material fails when the hoop stress reaches the tensile strength σtensile\sigma_{\text{tensile}}σtensile of the material, we can set σθ=σtensile\sigma_{\theta} = \sigma_{\text{tensile}}σθ=σtensile. Therefore, solving for P:
P=σtensile×t/2RP
This is the classical form of Barlow’s Formula. It estimates the internal pressure PPP at which the cylinder will fail due to hoop stress.
Final Form of Barlow’s Formula
Thus, the formula for the burst pressure PPP is:
P=2σtensilet/R
Where:
- P is the internal pressure that causes failure (burst pressure),
- σtensile is the material’s tensile strength,
- t is the wall thickness of the cylinder,
- R is the radius of the cylinder.
Barlow’s formula provides a simple and useful way to estimate the burst pressure of a thin-walled cylinder under internal pressure. However, it should be used with caution, as it assumes thin-walled conditions and does not account for many complex factors, such as material behavior at high temperatures or under fatigue loading. For more accurate results, especially in critical applications, more sophisticated analysis methods are recommended.

Essential FRP Pipe Code List for Engineers and Designers
FRP Pipe Burst Pressure Barlows
The frp pipe burst pressure barlows equation is one of the most important formulas used in the engineering and design of fiberglass-reinforced plastic (FRP) pipes. This formula helps professionals calculate the burst pressure of FRP pipes, ensuring they meet the necessary safety and performance standards. GangLong Fiberglass follows the guidelines of the frp pipe density burst pressure barlows equation to ensure that our FRP pipes can withstand the required pressures in industrial applications.
The frp pipe burst pressure barlows formula is a fundamental tool used by engineers and designers to calculate the burst pressure of fiberglass-reinforced plastic (FRP) pipes. This equation allows professionals to ensure that FRP pipes can safely withstand the internal pressures they are subjected to in various industrial environments. Whether in chemical processing, water treatment, or oil and gas applications, using the frp pipe burst pressure barlows formula is essential for determining the maximum internal pressure a pipe can handle before it fails.
What is FRP Pipe Burst Pressure Barlows Equation?
The frp pipe burst pressure barlows equation is a mathematical formula used to determine the burst pressure of cylindrical vessels, such as fiberglass tubes. The equation is widely used in the design of pipe systems to ensure that they can handle the internal pressures exerted by fluids or gases without failing. The formula is particularly important for FRP pipes because it helps ensure that the composite material’s strength is properly utilized while maintaining safety.
The equation for frp pipe burst pressure barlows is expressed as:
Burst Pressure = (2 * Tensile Strength * Wall Thickness) / Outside Diameter
In this formula:
- Burst Pressure refers to the maximum internal pressure the FRP pipe can withstand.
- Tensile Strength is the maximum stress that the FRP material can endure while being stretched or pulled before it fails.
- Wall Thickness is the thickness of the pipe wall, which significantly affects its ability to handle pressure.
- Outside Diameter is the external diameter of the pipe, influencing the pressure capacity based on pipe size.
This equation allows engineers to calculate the maximum internal pressure that a pipe can handle before it ruptures. The tensile strength of the FRP material, the wall thickness, and the outside diameter of the pipe are the critical factors in this calculation.
How About the Key Parameters in FRP Pipe Burst Pressure Barlows Equation?
There are three main parameters involved in the frp pipe burst pressure barlows equation: tensile strength, wall thickness, and outside diameter. Each of these parameters plays a vital role in determining the burst pressure of an FRP pipe.
- Tensile Strength: This refers to the maximum amount of stress that the FRP material can withstand while being stretched or pulled before it breaks. The tensile strength of FRP pipes varies depending on the resin and reinforcement materials used. GangLong Fiberglass ensures that all FRP pipes are made from materials that provide high tensile strength, improving their overall performance and durability.
- Wall Thickness: This parameter refers to the thickness of the FRP pipe wall. The thicker the wall, the more pressure the pipe can handle before bursting. Engineers must carefully select the appropriate wall thickness based on the intended application to ensure that the pipe can safely handle the internal pressures.
- Outside Diameter: The outside diameter of the FRP pipe is another crucial factor in determining burst pressure. Pipes with larger diameters tend to have lower burst pressures when compared to smaller pipes, assuming the wall thickness and material strength remain constant. Therefore, engineers must account for both the diameter and the wall thickness to determine the overall burst pressure.
What Are the Applications of the FRP Pipe Burst Pressure Barlows Equation?
The frp pipe burst pressure barlows equation is applied in various industrial sectors where FRP pipes are used, such as chemical processing, water treatment, oil and gas, and marine applications. In these industries, FRP pipes are often exposed to high internal pressures, making it essential to calculate their burst pressure to avoid catastrophic failures.
In chemical processing, for example, FRP pipes are used to transport corrosive fluids under pressure. The frp pipe burst pressure barlows equation helps engineers select pipes that can withstand the internal pressures generated by these fluids without compromising the structural integrity of the system. At GangLong Fiberglass, we apply this calculation to ensure that our FRP pipes are suitable for such demanding environments.
In water treatment plants, where FRP pipes are used to transport large volumes of water under pressure, the frp pipe burst pressure barlows equation helps engineers ensure that the pipes can handle the load without bursting. Similarly, in the oil and gas sector, FRP pipes are exposed to both high-pressure fluids and harsh environmental conditions, making the frp pipe burst pressure barlows calculation essential for safety and longevity.
How Does FRP Pipe Burst Pressure Barlows Calculation Help in Design?
The frp pipe burst pressure barlows calculation is a fundamental tool in the design process of FRP pipe systems. By using this equation, engineers can determine the appropriate pipe dimensions, material properties, and wall thickness to ensure that the pipe will not fail under the expected operating pressures.
This calculation helps to optimize the design by selecting materials with the appropriate tensile strength and determining the ideal wall thickness to withstand the internal pressures. Engineers use the frp pipe burst pressure barlows equation to balance cost and performance, ensuring that the pipe system is both safe and economical.
At GangLong Fiberglass, we integrate the frp pipe burst pressure barlows equation into our design process to provide customized solutions for our clients. Whether designing piping systems for industrial plants or municipal water projects, this calculation helps us select the right materials and dimensions to meet each client’s specific needs.
Maintenance and Safety Considerations in FRP Pipe Burst Pressure Barlows
The frp pipe burst pressure barlows equation is not only important for design but also plays a role in the maintenance and safety of existing FRP pipe systems. Periodic inspections and evaluations are necessary to ensure that the pipes are still capable of handling the internal pressures. Over time, factors such as wear, environmental degradation, and chemical exposure can reduce the tensile strength of the FRP material, making it more prone to bursting.
Engineers can use the frp pipe burst pressure barlows calculation to reassess the pipe’s current condition and determine whether any maintenance or replacements are required. This is especially important in industries where safety is a top priority, such as chemical processing or oil and gas.
GangLong Fiberglass offers inspection and maintenance services to ensure that FRP pipe systems continue to meet the necessary safety standards. Our team uses the frp pipe burst pressure barlows equation to verify that the pipes are still operating within safe pressure limits and provides recommendations for repairs or replacements if necessary.
FRP Pipe Burst Pressure Barlows and Future Developments
As the use of FRP pipes continues to expand across various industries, the frp pipe burst pressure barlows equation will remain a critical part of the design and safety processes. Advances in composite materials, such as the development of stronger resins and more durable reinforcement fibers, will likely lead to FRP pipes with higher burst pressures.
These advancements will enhance the performance of FRP pipes in extreme environments, making them even more reliable and durable. GangLong Fiberglass stays at the forefront of these developments, continuously improving our products to meet the ever-increasing demands of industrial applications.
How About the Importance of Tensile Strength in FRP Pipe Burst Pressure Barlows?
Tensile strength is a key factor in the frp pipe burst pressure barlows formula because it reflects the material’s ability to resist breaking under tension. For FRP pipes, tensile strength depends on the type of resin used and the reinforcing fibers that make up the composite material. FRP pipes made with high-tensile-strength resins, such as vinyl ester or epoxy resins, can typically withstand higher internal pressures.
The tensile strength value is usually provided by the material manufacturer and is measured in units such as megapascals (MPa) or pounds per square inch (psi). Higher tensile strength directly correlates to a higher burst pressure, assuming the same wall thickness and outside diameter. For example, an FRP pipe manufactured by GangLong Fiberglass using advanced resins will feature improved tensile strength, providing enhanced pressure resistance.
How Does Wall Thickness Affect FRP Pipe Burst Pressure Barlows?
Wall thickness is another critical component of the frp pipe burst pressure barlows formula. A thicker wall allows the FRP pipe to withstand greater internal pressures before it bursts. Engineers can adjust the wall thickness of a pipe to ensure that it meets the specific pressure requirements of its application.
For example, in a system where the internal pressure is high, engineers will specify FRP pipes with increased wall thickness to meet safety standards. Conversely, in lower-pressure applications, thinner walls might be acceptable. The frp pipe burst pressure barlows calculation helps engineers determine the minimum required wall thickness to ensure that the pipe can handle the expected pressure without failure.
At GangLong Fiberglass, we offer a range of FRP pipes with varying wall thicknesses to accommodate different pressure requirements, all designed according to the frp pipe burst pressure barlows guidelines.
How About the Role of Outside Diameter in FRP Pipe Burst Pressure Barlows?
The outside diameter of an FRP pipe is the final variable in the frp pipe burst pressure barlows equation. Larger-diameter pipes typically have lower burst pressure capacities compared to smaller-diameter pipes of the same wall thickness. This is because the larger surface area of the pipe’s internal wall is exposed to more pressure, increasing the likelihood of failure if the pipe is not designed appropriately.
For this reason, engineers must consider both the outside diameter and the wall thickness when selecting the correct pipe for an application. A careful balance between these two parameters ensures that the FRP pipe will be able to handle the desired pressures safely.
GangLong Fiberglass provides FRP pipes in various diameters, and our team uses the frp pipe burst pressure barlows equation to help clients choose the appropriate pipe size for their specific pressure requirements.
Practical Example of FRP Pipe Burst Pressure Calculation with Barlow’s Formula
To better understand how the frp pipe burst pressure barlows formula works, let’s walk through a real-world example of calculating the burst pressure of an FRP pipe. For this example, we will assume the following values for the FRP pipe:
- Tensile Strength: 150 MPa
- Wall Thickness: 10 mm
- Outside Diameter: 200 mm
Step 1: First, identify the key parameters from the equation:
- Tensile Strength = 150 MPa
- Wall Thickness = 10 mm (0.01 m)
- Outside Diameter = 200 mm (0.2 m)
Step 2: Plug these values into the frp pipe burst pressure barlows formula:
Burst Pressure = (2 × 150 MPa × 0.01 m) ÷ 0.2 m
Step 3: Perform the calculation:
Burst Pressure = (300 MPa × 0.01 m) ÷ 0.2 m
Burst Pressure = 3 MPa ÷ 0.2 m
Burst Pressure = 15 MPa
In this example, the FRP pipe can withstand an internal pressure of 15 MPa before it bursts. This calculation is critical for ensuring the pipe can handle the pressures of the system it is designed for. GangLong Fiberglass uses similar calculations to confirm that each of our FRP pipes meets the necessary pressure ratings for its intended application.
How to Use FRP Pipe Burst Pressure Barlows in Design?
The frp pipe burst pressure barlows formula plays a crucial role in the design phase of FRP pipe systems. By calculating the burst pressure, engineers can determine the minimum wall thickness, material strength, and pipe diameter required for safe operation under the expected internal pressures. This ensures that the pipes are neither over-designed (leading to unnecessary costs) nor under-designed (resulting in safety risks).

How to calculate frp pipe friction factor for efficient flow
Allowable Stress and Its Role in FRP Pipe Burst Pressure
Allowable stress refers to the maximum stress a material can endure without failing. In the frp pipe burst pressure barlows formula, the allowable stress of the material is a key factor in determining the pipe’s overall burst pressure. The higher the allowable stress, the more pressure the pipe can handle.
FRP materials have varying levels of allowable stress depending on their composition and the manufacturing process. In the case of GangLong Fiberglass pipes, allowable stress values are carefully calculated based on rigorous testing to ensure that the pipes can perform under various pressure conditions. Engineers must consider this factor when designing piping systems, as underestimating the allowable stress could lead to premature pipe failure.
The Role of Outside Diameter in Barlow’s Formula for FRP Pipes
The outside diameter of a pipe also plays a crucial role in determining burst pressure, according to the frp pipe burst pressure barlows formula. The larger the diameter, the lower the burst pressure. This inverse relationship means that larger pipes may require thicker walls or higher allowable stress ratings to maintain the same level of pressure tolerance as smaller pipes.
For example, a GangLong Fiberglass pipe with an outside diameter of 24 inches will have a significantly lower burst pressure compared to a pipe with an outside diameter of 12 inches, assuming all other factors remain the same. When designing piping systems, it’s essential to balance the pipe’s diameter with the material’s strength and the expected pressure loads.
Practical Application of Barlow’s Formula for FRP Pipe Systems
The frp pipe burst pressure barlows formula is not only useful in theoretical calculations but also has practical applications in the field. Engineers and designers use this formula to ensure that their FRP piping systems are safe, reliable, and able to withstand the operational pressures they will face.
One practical example is in the water treatment industry, where FRP pipes are commonly used to transport high-pressure water. A GangLong Fiberglass FRP pipe installed in such a system must be able to handle the continuous pressure without failing. By using Barlow’s Formula, engineers can select the appropriate pipe dimensions and material strength to prevent failures.
Another application is in chemical processing plants, where the risk of pipe failure due to high pressure could lead to hazardous leaks. The frp pipe burst pressure barlows formula ensures that pipes are designed with the correct safety margins, protecting both the equipment and the people working around it.
Using a Calculator for FRP Pipe Burst Pressure Barlow’s Formula
Many industry professionals rely on online calculators to quickly determine burst pressure for FRP pipes. These calculators use the frp pipe burst pressure barlows formula to provide accurate results based on the inputted values for wall thickness, allowable stress, and outside diameter.
GangLong Fiberglass, for instance, provides a simple interface where users can enter their pipe specifications and receive instant calculations of the burst pressure. This tool is particularly useful for engineers who need to make quick decisions about the design and implementation of piping systems.
By entering values such as wall thickness, allowable stress, and outside diameter into the calculator, users can easily compare different FRP pipe options and select the one that best meets their needs. While these calculators are a convenient tool, it’s important to remember that the results should be verified by an experienced engineer before implementation.
Practical Example of Using Barlow’s Formula for FRP Pipes
Consider a GangLong Fiberglass FRP pipe used in an oil refinery. The pipe has a wall thickness of 0.5 inches, an allowable stress of 8,000 psi, and an outside diameter of 18 inches. Using the frp pipe burst pressure barlows formula:
P = (2 * 0.5 * 8,000) / 18
P = 444.44 psi
This calculation shows that the pipe can safely handle up to 444.44 psi of internal pressure. Such calculations are essential for ensuring that the pipe can perform under the specific conditions of an oil refinery, where high pressures are common.
FRP Pipe Fire Rating: What Every Industry Needs to Know
Limitations of FRP Pipe Burst Pressure with Barlows
Barlow’s formula is a widely used empirical formula in engineering to estimate the bursting pressure of thin-walled cylinders. It is specifically applied to pipes and tubes under internal pressure, providing a relationship between the internal pressure, the material strength, and the geometric dimensions of the cylinder. While it is a helpful tool, there are several limitations to Barlow’s formula that engineers and designers need to be aware of:
Assumption of Thin-Walled Condition
Barlow’s formula is derived under the assumption that the wall thickness of the pipe or cylinder is much smaller than its internal radius. This is known as the “thin-walled assumption.” When the wall thickness becomes comparable to the radius of the cylinder, the formula becomes inaccurate. In such cases, the stress distribution across the thickness of the wall is no longer uniform, and more complex models (such as Lamé’s equations) are required.
Material Properties
The formula assumes that the material of the cylinder is homogeneous and isotropic, meaning it has uniform properties in all directions. In reality, most materials have some degree of anisotropy or inhomogeneity, especially composite materials or metals with varying grain structures. Additionally, Barlow’s formula assumes that the material is perfectly elastic, not taking into account plastic deformation or material failure due to fatigue or creep over time.
No Consideration for External Forces
Barlow’s formula is based on the internal pressure acting on a cylinder, and it does not account for external loads or forces, such as bending, torsion, or axial loads. In real-world applications, pipes and tubes often experience combined stresses due to both internal pressure and external forces, which can affect the overall strength and safety of the structure. For more complex loading conditions, additional analyses are necessary.
Stress Concentrations and Geometrical Irregularities
The formula assumes a perfect, smooth, and regular cylindrical shape. In practice, pipes and tubes often have imperfections, welds, or geometrical irregularities that can create stress concentrations. These local high-stress areas are not considered in Barlow’s formula, which can lead to overestimating the safety of a pipe or tube. Design codes often incorporate safety factors to account for such issues, but these are not explicitly handled by the formula itself.
Temperature Effects
Barlow’s formula does not account for the effects of temperature on material strength and expansion. Materials typically weaken at higher temperatures, and the strength of the material decreases. Similarly, at low temperatures, materials might become brittle. The formula assumes that the material properties are constant and unaffected by temperature, which is not always the case in real-world applications, especially in industries where pipes may be exposed to extreme temperatures, such as in power plants or chemical processing.
Does Not Account for Fatigue or Cyclic Loading
The formula is based on the assumption of a static internal pressure. However, in many applications, the internal pressure can fluctuate or vary over time, leading to fatigue damage in the material. Barlow’s formula does not consider fatigue life or the effects of cyclic loading, which is a critical factor in the design of pressure vessels, pipelines, and other components exposed to repeated or fluctuating pressures.
Safety Margin and Over-simplification
Barlow’s formula is a simplified empirical equation, which may not capture all the complexities of material behavior and load conditions. While it provides a quick estimate, it is not always reliable for precise, high-stakes designs. Engineers typically apply a safety margin to account for uncertainties in material properties, manufacturing defects, or unexpected loading conditions. The lack of these considerations in Barlow’s formula can lead to designs that are overly simplistic and might not meet all safety standards, especially for critical applications.
Limited Applicability to Certain Pipe Materials
The formula is more suited for cylindrical structures made from ductile materials like steel or aluminum, but it may not provide accurate results for more brittle or complex materials, such as certain ceramics or composites. These materials behave differently under stress and might fail in ways that Barlow’s formula cannot predict, particularly when they are subjected to sudden impacts or localized stress risers.
While Barlow’s formula is a valuable tool for the preliminary design and analysis of pressure vessels and pipes under internal pressure, its limitations mean that it should be used with caution. For accurate and safe designs, it is essential to consider the specific conditions of the application, including material properties, wall thickness, external forces, and fatigue effects. More advanced analytical methods and safety codes should be used in conjunction with Barlow’s formula for critical applications.
FAQs about Frp Pipe Burst Pressure Barlows
The ASME (American Society of Mechanical Engineers) provides a specific formula for calculating the burst pressure of pipes, especially in high-stress environments like pressure vessels. The ASME formula considers the material’s allowable stress, the pipe’s dimensions, and design factors to ensure safety and compliance with industry standards. The formula for ASME burst pressure is:
Burst Pressure = (2 * Allowable Stress * Wall Thickness) / (Outside Diameter – Wall Thickness)
In this formula, the allowable stress is the maximum stress the material can withstand as per ASME standards, and the wall thickness and outside diameter refer to the pipe’s physical dimensions. This formula is more conservative than Barlow’s Law, as it includes safety factors to prevent material failure under unexpected conditions. Adhering to the ASME standards ensures that pipes operate safely within the specified pressure limits.
Barlow’s Law is a formula used to calculate the burst pressure of cylindrical objects, such as pipes, based on the material strength, wall thickness, and diameter. The law is especially useful in industries dealing with pressurized pipelines. The formula is:
Burst Pressure = (2 * Tensile Strength * Wall Thickness) / Outside Diameter
Barlow’s Law helps engineers and designers understand the maximum pressure a pipe can handle before it ruptures. The tensile strength represents the maximum stress the material can withstand, while the wall thickness and outside diameter are specific to the pipe dimensions. This law assumes that the material behaves linearly under stress and that the pipe is subjected to internal pressure uniformly. While Barlow’s Law provides an essential calculation for designing safe pressure systems, safety factors are often applied in practical applications to account for unforeseen stresses or imperfections in materials.
Burst pressure is the maximum internal pressure that a pipe can withstand before it fails or bursts. To calculate the burst pressure of a pipe, several factors need to be considered, including the pipe’s material strength, wall thickness, and diameter. A common approach to calculating burst pressure is by using Barlow’s Law, which provides a formula for determining the burst pressure of cylindrical structures like pipes. The formula is:
Burst Pressure = (2 * Tensile Strength * Wall Thickness) / Outside Diameter
In this equation, the tensile strength is the maximum stress the material can handle, the wall thickness is the thickness of the pipe wall, and the outside diameter is the pipe’s outer diameter. It is important to note that different materials and standards may require additional factors for accuracy.
The maximum pressure for an FRP (Fiber Reinforced Plastic) pipe depends on the material composition, design, and operating conditions. Generally, FRP pipes can withstand pressures ranging from 150 to 2,000 psi, with some high-performance grades designed for even higher pressures. For example, FRP pipes made from thermoset resins like vinyl ester or epoxy can endure greater stress than those made from polyester resins. The pressure rating also depends on the pipe’s diameter, wall thickness, and reinforcement material, so it’s essential to consult manufacturer specifications for precise ratings in specific applications.
FRP pipes have several potential problems, including susceptibility to damage from impact or abrasion, and issues related to long-term fatigue and stress. UV degradation can weaken the material when exposed to sunlight over time, unless UV-resistant coatings are applied. Additionally, chemical compatibility with certain fluids is a concern, as certain chemicals can cause resin degradation. The pipes may also be prone to leakage if not properly installed or if subjected to extreme thermal cycling. Finally, the cost of manufacturing and repair can be higher than other materials, depending on the specific application.
The design pressure for an FRP pipe is determined based on factors like material properties, pipe dimensions, and intended usage conditions. Generally, FRP pipe design pressure is calculated using a combination of the material’s ultimate tensile strength, the safety factor, and the pipe’s wall thickness. A typical design pressure for FRP pipes may range from 150 psi to 1,500 psi, depending on the pipe’s application. The design pressure is typically lower than the burst pressure to ensure a safety margin, preventing failure during operational conditions. Each manufacturer may also provide their own design guidelines.
Bursting pressure is calculated using a formula that incorporates the material strength, pipe dimensions, and wall thickness. The general formula is:
P{burst} = (2×S×t)/D
Where:
Pburst is the burst pressure,
S is the material’s tensile strength,
t is the pipe wall thickness,
D is the pipe diameter.
This formula assumes that the pipe is perfectly cylindrical and the stress distribution is uniform. In practice, safety factors and environmental conditions are also considered to determine the maximum safe burst pressure.

As the editor of GangLong Fiberglass, I have years of experience and in-depth research, focusing on cable tray products, fiberglass solutions, and grille systems. I incorporate years of industry insights and practical experience into every content, committed to promoting the progress of the industry. At GangLong Fiberglass, my commitment is reflected in every product, from innovative cable trays to durable fiberglass solutions and sturdy grille systems. As an authoritative voice in the industry, my goal is to provide valuable information to professionals and businesses and promote forward-looking solutions.